How free is a free electron?
We know from history: Freedom is something worth fighting for, freedom is the word that has always been capitalized today and in the past.
Anyway, when one is free he can do anything, go where he/she wants.
This can apply to a free electron, for example – or not? Are free electrons really free?
They are those electrons that, for example, can move freely in a metallic lattice driven by the smallest electric field, since they are not assigned to and bound to an atomic nucleus. If that’s not enviable enough, let’s take a look at our most recent electricity bill and see that electrons are very well paid on the top of it.
Somehow, all electrons are famously negative. But what do they have to complain about? Is a free electron really as free as its name suggests?
I did a little research on how far they can travel if they feel like going on a wild adventure. We learn in school that electrical energy travels along the wire at the speed of light. If I turn on a switch on a long pair of wires in Budapest, while there is a Lamp on the other end of the wires say, on my favorite beach on the Adriatic, approx. after 2 milliseconds, i.e. two thousandths of a second, the current appears, the lamp lights up. If this is not freedom, then nothing else is, because I make the same trip in the best case with 6 hours of bumping in the car.
But let’s stop for a moment! No one said that the electron from the switch in Budapest would personally reach the other end of the wire at the speed of light in, say, Rovinj in no time. No, the electrical interaction between the electrons, actually the effect of repulsion, is transmitted, but not the electron itself. The poor electron may just push its energy further down the wire without travelling any remarkable distance.
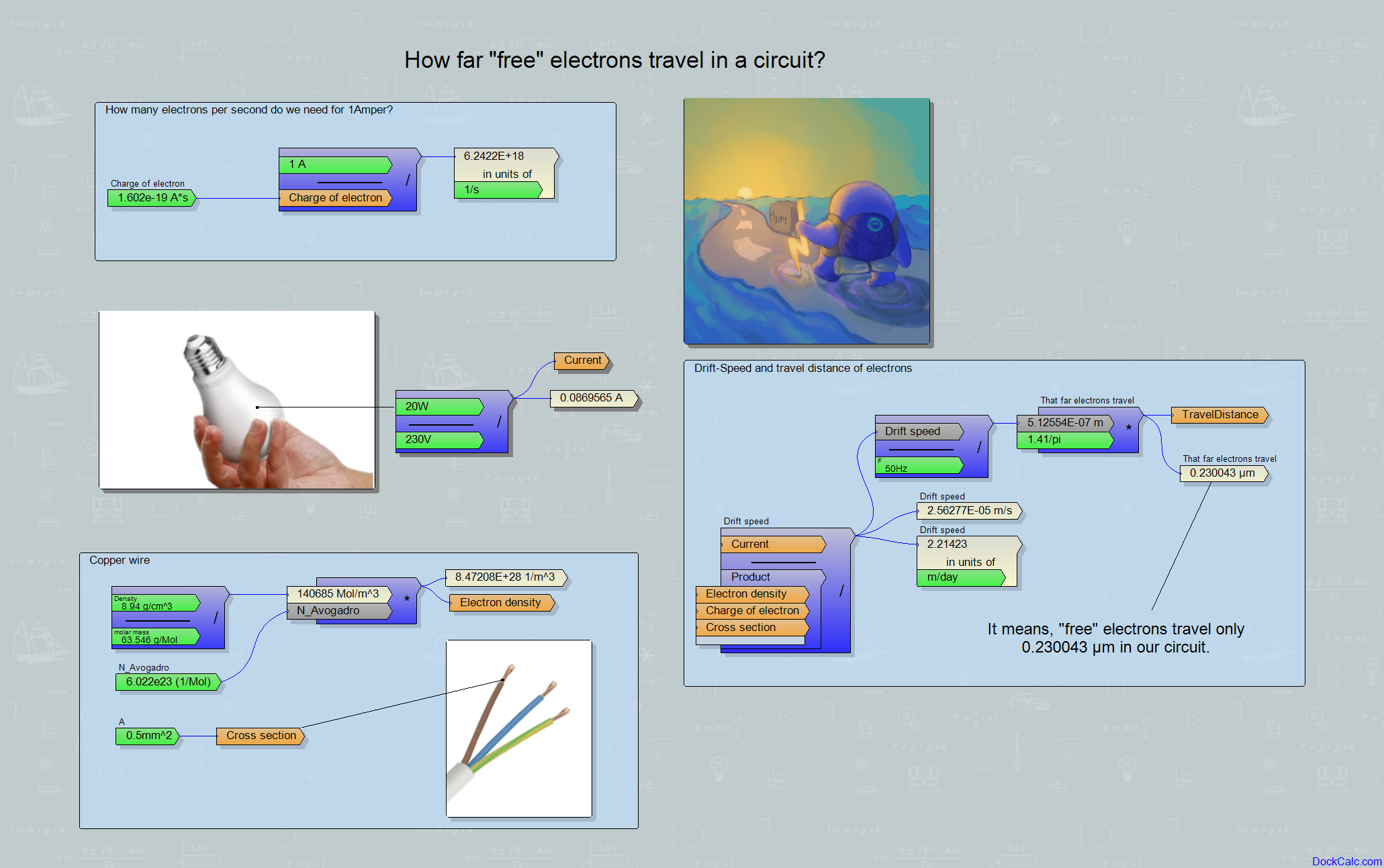
But such a thing can be calculated, since we learned that a Coulomb charge passes through a given cross-section in one second for a current of one Ampere. You only need to know how densely the electrons are in the wire and then you can find out how far they have to move and how fast they move for a given current. Wikipedia says that an electron has a charge of −1.602*10−19 Coulombs. So one Ampere current means that roughly 6.2422*1018 electrons pass somewhere in one second. Obviously, the thinner the wire, the faster they have to go for this, but if the wire is too thin, it heats up due to the resistance, so we cannot send much current through a thin wire. I must note here that the life of an electron is greatly simplified here and unfortunately it cannot be observed with the naked eye (not even with a microscope), and those who have held electrons in their hands have probably gained an eternal memory, but still do not fully understand them.
The subject of my reflection now is only the average speed of electrons in a conductor, which is called the drift velocity, so I want to calculate this specific value. We have just seen how many electrons a given current means per second, and if we knew how densely the electrons populate the conductor, then their speed can simply be calculated from there:
drift velocity=Current/(electron density*electron charge*wire cross section)
The electron density can cause a bit of a headache, although, if we calculate with copper (the wires are often made of it), then by combining the table of materials and chemistry memories, we get data such as the density of copper (8.94 g/cm3), its molar mass (63.546 g/Mol) which are divided into a value of 140685 Mol/m3, which, multiplied by Avogadro’s number (6.022*1023), gives 8.5*1028 atoms/m3, which tells, how many atoms are in one cubic meter of copper. Copper has one free electron per atom (each atom “throws one electron into the common”), so the density of free electrons is the same: 8.5*1028 electrons/m3. From the 28 zeroes, it can be felt that a lot of electrons will flow through a conductor at very low speeds. Let’s look at a practical example: a 20-watt LED lamp powered by mains voltage (230V) consumes only 0.087 A of current. The usual wire cross-sections in the European households are around 0.5 mm2 and 1.5 mm2, let’s choose a thin one so that our electrons can travel further: 0.5 mm2!
By substituting everything into the formula written above, the drift velocity is given as follows:
drift velocity=0.087A/(8.5*1028 electrons/m3*1.602*10-19 Coulomb*0.5 mm2) = 2.563*10-5m/s
that is, no more than 2,215 meters per day.
And only now comes the really disappointing fact:
we run our machines and lights on alternating current. Therefore, our electron not only moves with minimal speed, but that also just back and forth. The distance along which an electron “wanders” is thus limited to the range of micrometers. Of course, out of sheer compassion, we can give our electrons some adventure by using thinner wires and more powerful machines, increasing the living space of our negative friends even to a few millimeters, although safety and economy considerations do not recommend such a thing. Let us comfort ourselves with the fact that our electrons will probably be by our side for many ten years, serve us and it is really possible that the same electrons that are currently offering brightness for our child’s preparation for the high-school diploma are the same ones that have witnessed the first steps of the child years earlier.
So let’s be careful with them and let some of them climb the Christmas tree in a chain of lights so that they can celebrate the coming Christmas together with us!
I recommend performing the calculations in this article at home only to strong-minded, well-educated professionals, or to those who have already downloaded the DockCalc Calculator software. With DockCalc it is as simple as this: